Achieving independent and fluent calculation strategy selection

Since we wrote and launched Number Sense Maths, we’ve spent a lot of time talking to people about derived fact strategies, and introducing people to this approach for teaching number facts to fluency.  NSM teaches 12 strategies through the course of the programme that collectively lead to fluency in all of the addition and subtraction grid facts.  This blog post describes what happens once all the strategies have been taught so that children achieve true factual fluency.

As you teach each strategy, you will see children fairly quickly becoming confident in applying them.  Near doubles, for example, is a strategy that quite a lot of children just don’t start to use without explicit teaching, but our experience is that once it is taught, the near doubles strategy is intuitive and easily applied by the vast majority of children.

But solving 3 + 4 by using near doubles when children are already primed to think about the strategy is very different from solving 3 + 4 using near doubles once it is presented among calculations that call for a range of strategies e.g. 8 – 6, 2 + 5, 6 + 3.  So once we have taught a strategy and children are able to use it with facts that correspond to that strategy, we need to provide an opportunity to practice selecting the correct strategy AND executing it.

This idea - teaching something discretely, and then setting up situations where children need to use this new skill alongside other skills and knowledge - is something we do all the time as teachers.  In PE for example, we may teach a one-legged static balance, and teach children to keep their head up and still, their tummy tight and their back straight.  But once the children have learnt this and other skills (such as particular jumps and correct landings) in isolation, we then challenge them to pull all of those together into a sequence.  We provide practice until the children can independently perform all of those moves as they need them, prompting as necessary (“Remember, tummy tight”).  As teachers we are very skilled at scaffolding this move to independent application of a new skill, so we just need to apply these pedagogical skills to number facts. 

So what does it take for us to be effective prompters of number fact strategies?

• Firstly, we need to know a strategy for each fact as an alternative to counting on or counting back. What do we say, for example, if a child asks us “How do we do 9 – 7?”?  Well in Number Sense Maths this fact is covered by our ‘number neighbours’ strategy: next door odd numbers have a difference of 2.  Knowing a strategy for each fact is something you will need to teach yourself to become fluent in.  You can practice this by going to our strategy selection books in Stage 3 and Stage 5, and printing out the fact cards in the top section of that book and then testing yourself.  For any you aren’t sure of, our coloured mapping grid will help you identify a strategy. Or you can play the animations in the strategy selection books at the end of Stages 3 and 5 (play them at double speed by clicking on the settings cogs) and practice recalling the strategy this way. 
• Secondly, we need to decide how to prompt the child who asks for help with 9 – 7. We need to consider the appropriate level of scaffold – like Goldilocks, we are aiming for not too much and not too little, but the ‘just right’.  And the earlier children are in selecting the strategies, the more scaffolding this ‘just right’ will involve.  If I was prompting a child to solve 9 – 7, here’s what that would look like:
  • When a child is near to independence I would say, “Look carefully. It’s 9 and 7.  There’s something special about those two numbers together – can you think what it is?”
  • Or, to introduce a bit more support, “I am looking at 9 and 7, and I have noticed that they are next door odds. Can you remember what we have learnt about next door odds?” 
  • Or I could add a sentence starter: “Next door number neighbours have a difference of 1, and next door odds have….”
  • And if a child was still unsure, that is a sign to me that I need to go back to the models used in teaching to show the child that next door odds have a difference of 2, and expose why this is.

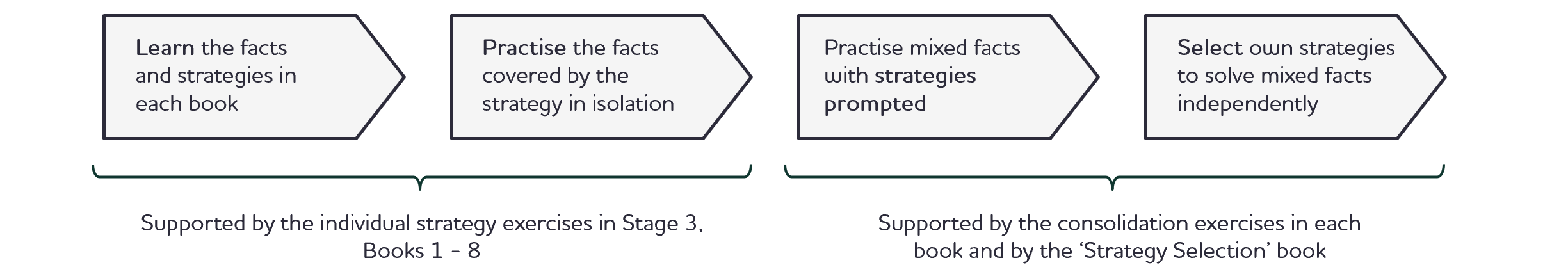
This gradual withdrawal of support until children can select the strategies for themselves is just as important a part of the teaching sequence as the initial teaching of the strategies.  It is what leads to fluency, where children can select AND apply their own strategies. We suggest that you build in about 4 weeks at the end of Stage 3 and Stage 5 for this explicit strategy selection teaching.  After this, you will of course still provide the occasional prompt as and when children need it.

At first you will be a novice at this, but with practice and reflection you will become an expert at it and NSM has tools to support both you and the children with this.

• In Stages 3 and 5 where the main strategy teaching takes place, the two exercises at the end of each book combine calculating new facts with previously learnt ones.
• The strategy selection books at the end of Stages 3 and 5 have a range of exercises and animations, with prompts and models progressively withdrawn, to help the children both select and apply strategies.

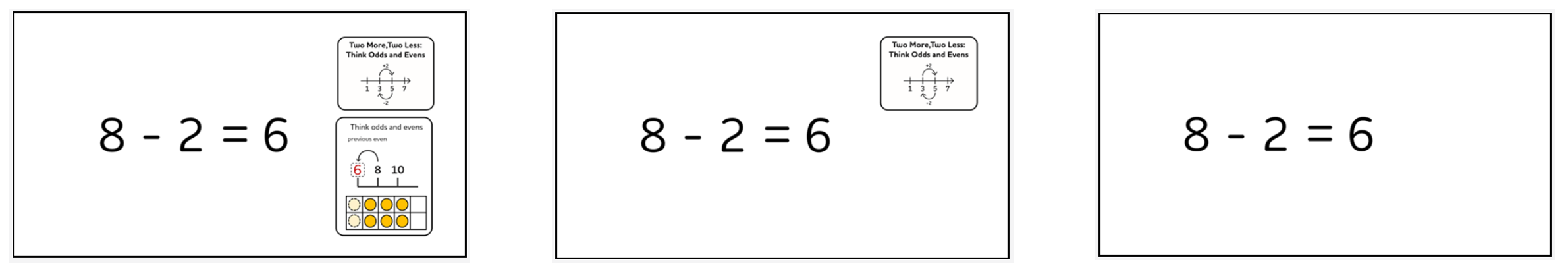
Gradual withdrawal of support in Stage 3 Book 9 strategy selection animations: strategy prompt and model of structure, then just strategy prompt, then no prompt

Alongside the PE analogy, we’ve recently we’ve been thinking about the analogy of learning to drive.  Learning to reverse around a corner having just been taught to do so is one thing, but then we have to be able to interchange between this and other manoeuvres – gear changes, hill starts, parallel parking and so on – moving seamlessly between one and the next as and when we need it.  A good instructor will moderate the amount of support and prompt they provide as we pull those manoeuvres together, from a full ‘talk through’ to a one word nudge: ‘clutch’. And the same idea applies to number facts. This teaching, prompting, and gradual withdrawal of support leads to fluent calculation of addition and subtraction facts for a child to draw on whenever they need them on their journey through mathematics. 

Back to blog